Going full circle completing the square

I usually use the last six week of the school year in geometry to teach circles: Arc lengths, chords, secants, tangents, etc. Which I have outlined in the Geometry Planning Guide

The following school year students begin Algebra 2 and promptly forget these properties of circles. They also start the new school year frustrated and overwhelmed because they forgot all about the quadratics they learned in Algebra 1 during their year in geometry.

This school year I decided to make some changes to the last unit in order to set students up to be more successful in the transition from Geometry to Algebra 2:

1)   I began with a modified version of Fawn’s task: When I got them to beg

I distributed an index card to each student and gave them students 5 minutes to make a beautiful work of art on the card. When time was up, students turned in their art, I shuffled the cards and redistributed them to the class.

I cut orange paper to the same size as 3×5 inch index cards, and told the students it was “Real Gold” it was very expensive and we could not waste any of it (they referred to orange paper as “Gold” for the rest of the school year). They had to try to make a frame for the art and tape it down to the assignment sheet that I distributed to them with an explanation of their thinking and strategy.

It worked. They begged me to help them find a better way to complete this task.

2)  Complete the Square with algebra tiles.

I used this activity from Salt Lake City Schools to guide student thinking. Students worked in small groups to create squares using algebra tiles and relating the squared and factored forms of perfect square quadratic equations. We referred back to this task often throughout the unit.

3) Solving equations by Completing the square notes and practice

I used Sarah Hagan’s foldable for completing the square. The following day I planned a basic practice sheet for students to just build fluency with solving equations by completing the square, but it was so nice out, we decided to do this on the sidewalk instead. (Note: Always keep sidewalk chalk in your classroom for beautiful day emergencies)

4) Applications of completing the square

I returned students framed art task from day 1 above, and they wrote and equation and  found the appropriate frame size using completing the square, then measured their estimated solution and reflected on their work.

Next we completed the Shell center task: Cutting Corners

5) Connecting Quadratic equations to Parabolas

I printed and laminated sets of the domino cards from the Shell Center task: Representing Quadratic Functions Graphically and students completed the loop and filled in the blanks. They then were able to summarize the relationship between standard, vertex and factored form of a quadratic equation and understand what each of the forms of the equation illustrated about it’s graph.

The next day, students worked on the Desmos Activity: Match My Parabola. I was able to pause and pace this activity as needed and monitor students understanding in order to support students understanding of the various forms of a quadratic equation.

6) Converting a quadratic equation between vertex, standard and factored forms

Now that students understood the usefulness of the various forms of quadratic equations, they wanted to be able to convert a quadratic equation between the various forms:

This slideshow requires JavaScript.

6) Develop the equation of a circle

I started with this question:

There were a few students who shouted out “Pythagorean Theorem!” and students realized they could construct a right triangle and find the length of the hypotenuse/radius.

Next, I gave students individual whiteboards and asked them to draw a circle centered at (0,0) with a radius of 5. Then I asked them to name coordinates of points that they know for sure were on the circle and I would have them explain how they knew. Eventually we identified 12 points on the circle.

After this, I asked students what the relationship was between the x and y coordinates on the circle. Most groups were able to explain that they were all related by the Pythagorean theorem because x² + y² = 5².

At this point, I had students complete the first side of the “Going Round in Circles” sheet from this Shell Center lesson in order to see how well students understood the discussion and to see how they could apply their learning.

The next class period began by asking students to find the radius of a circle given the coordinates of the center and a point:

The vertical height of the right triangle should be 12, not 13! This was corrected during the class discussion.

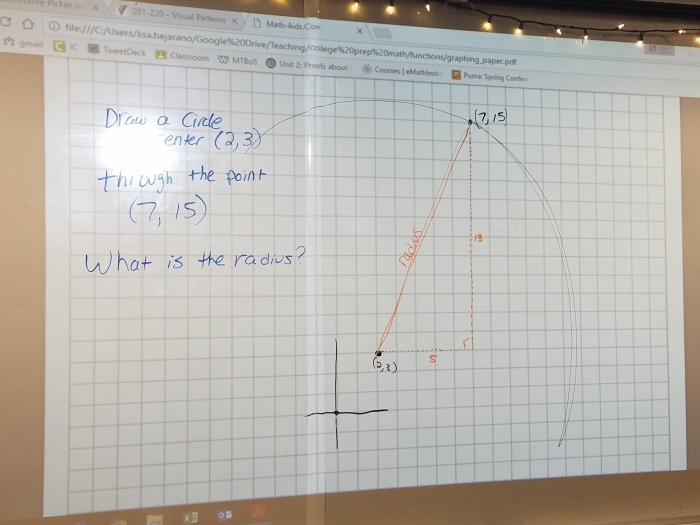
We used this to determine the radius of this circle as (7 – 2)² + (15 – 3)² = 13²

After further discussion, students were able to generalize the equation of a circle centered at (h, k) to be:  (x – h)² + (y – k)² = r²

Then students worked in small groups on the vertical white boards to complete the task included in the Shell center lesson: Sorting Equations of Circles 1

I printed and laminated the cards to make them easier to use on vertical dry erase boards and for facilitate discussion.

Finally, students completed the “Going Round in Circles, Revisited” sheet included in the lesson linked above.

7) Find the center and radius of a circle by completing the square

I created a foldable for students to summarize the equation of a circle that included examples of using completing the square to put an equation of a circle into a standard form. We followed this with additional practice.

<foldable below should be printed on legal sized paper> 

Every time we referred back to completing the square I made visual diagrams connecting it back to their initial development of their understanding of how to complete a square. I never stated any shortcut like, “just divide by 2 and square it.”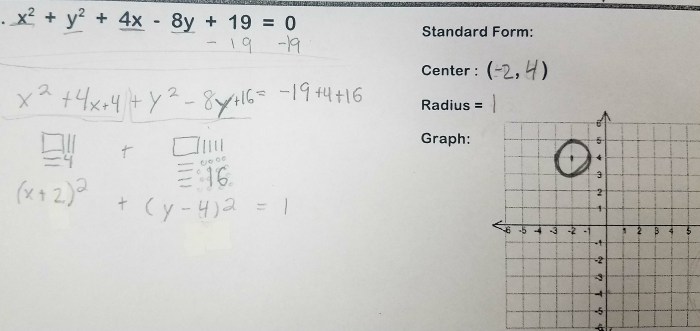  Students developed a genuine understanding of the process, which will hopefully lead them to increased success as they begin algebra 2 next school year.

Woot!!

Rolling Cups: Modeling in Geometry

The Rolling Cups task from the Shell Center is a perfect geometry modeling project. I use some form of this task every year for the last three days of the first semester as a final project. This task incorporates constructions, similarity, functions and modeling while pre-assessing students readiness for next semester’s content: solids and circles.

Here is this link to the Formative Assessment Lesson

I’ve discovered that this activity is most effective when I have students produce something each of the 50 minute class periods. Over the years, I have been collecting a wide variety of cups from Goodwill, which I keep in a giant tote in the basement of my school.

With finals complete and students’ motivation dwindling, Rolling Cups is the perfect way to make the most of the days right before break. I move all of the chairs and desks out of the way that we have a big open space in the middle of the class. I also make sure students can easily access the dry erase boards on all of my walls to encourage teamwork and collaboration (more on vertical dry erase boards).

Here is how I break up this activity-

Day 1: Experiment

Here are 4 cups. What will happen when I roll them? Which one will make the biggest circle? Which one will make the smallest? Try it.

I hand each student a different cup and this sheet below to guide their thinking and keep them on task, and then I get out of the way.

At some point during this class period I also show students the Rolling Cup Calculator. I put a link in Google Classroom for easy access. Most students use it on their cell phones to try to find patterns.

Day 2: Develop an equation

This is the formula derivation day. I start by not mentioning the cups at all and just playing a quick whiteboard game reviewing similarity.

During this task, I usually have a few students suddenly yell out:

“This is the cup thing!”…and then start sketching cups on their whiteboards and they begin to use similar triangles to determine the roll radius.

Here is a discussion I had with a student, trying to support their thinking:

Other students look at the first group like they are crazy and we just carry on.

Then I have a few students summarize what they’ve noticed from the previous class.  Next I hand out this sheet: side 1 is the original task and side 2 is for students to write a few sentences summarizing their findings, and score themselves. My school has been working to develop a structure and rubric to elicit quality student writing about mathematics. Below is the current format.

As I present this sheet to students and summarize the expectations for the day, I also tell them that I made a deal with another teacher on Twitter and that I will be scanning their work and sending it to this teacher in Ohio.

I make a big deal about them not writing their names on the back of the sheet where they describe their thinking because that is the side I am going to send to Ohio and I want to preserve their anonymity. In return, I explain, the class in Ohio will be sending me their work, which we will look at tomorrow.

The quality of students work is so much better when they think it will be analyzed by someone else. Of course, this is a big fat lie. I don’t have any plan to send their work to another teacher. But they do so well with this added piece of motivation.

Day 3: Critique other students work

This day goes pretty much as described in the original task. Students review the included samples of other students work and analyze it answering the well written questions from the original task linked above.

It is fun for students to see that other students in other parts of the country approached the problem the way they did. They get excited and genuinely interested. They begin to reflect on their approach and compare it to the student work provided. You can see their confidence grow a little when they recognize that their (alternative school) work is just as good, if not better than the work of typical students in Ohio.

Painless Proofs!

Last year I didn’t really teach proofs in geometry. It seemed so procedural and I knew it would take time away from more productive problem solving tasks.

I felt guilty for not including proofs in enough detail and I decided that this year I was going to teach it well.

Here is what I did:

1. Our unit began with the Shell Center task: Evaluating Conditions for Congruency, I don’t always follow these lessons exactly as described, but we focused on the Must the Two Triangles be Congruent? part of this lesson. I found that this activity is much smoother and more effective when using AngLegs instead of trying to draw each triangle (described here and here).
2. After this task we formalize our findings in our notebooks documenting which combinations guarantee congruent triangles [SAS, SSS, ASA, AAS] and which do not [SSA, AAA].
3. Next students practice determining if pairs of triangles must be congruent based on the information given. I am careful to include a few with overlapping triangles and triangles who share a side or contain vertical angles to generate observations and class discussions. At this point I address the reflexive property and vertical angles as reasons sides or angles may be congruent. I follow up any student observations of congruency with “How do you know?” or “Explain why you decided these two angles must be the same.”
4. Review definitions of midpoint, bisector, perpendicular, then a few images where I ask if you are given this information, what can you conclude is congruent. See the interactive notes here. This is the day students complete their first few **Really Basic** congruent triangle proofs.
5. Prior to this class I printed Proof Blocks on colored paper and laminated them, then I used masking tape to affix them to  whiteboards. Once class began I randomly assign student pairs and had them work on a wall mounted white board (Vertical non-permanent surface) with a set of Proof Blocks at each work space.

.

Next I project a proof and students copied the image onto the whiteboard, then marked the givens, saw what else they could determine was congruent, and decided if they had enough information to conclude and prove that the triangles must be congruent.

In order to make sure all students got timely feedback and to hold them accountable, I gave each group an index card and when they finished a proof I would check it. Once it was done well, they got a stamp on their card. At the end of class they turned in their index card with their names & stamps on it, similar to the review activity I described earlier.

I returned to this format with the proof blocks and different pairs about once per week after learning and incorporating new skills. The second half of the slides above were from a day after learning and practicing angles formed by parallel lines & a traversal. I intend to create new “blocks” as we advance through the school year. Next week students will prove that all triangles have a sum of 180 degrees and that base angles of isosceles triangles are congruent using the same format.

With students standing at white boards, they can glance around and see their peers work easily, they are more likely to collaborate, and the whiteboard & Proof Blocks make it much easier to adjust their work when there are any corrections required.

I just graded students quizzes over this unit and it is the first time in my 12 year teaching history that they did very well on the first attempt!