Planning content for College Prep Math

I teach a single semester math class that students choose to take in an effort to avoid taking algebra 2. The class is primarily seniors whose highest level of math is geometry, and they plan to join the military or work and take a few classes at a time from our local community college.

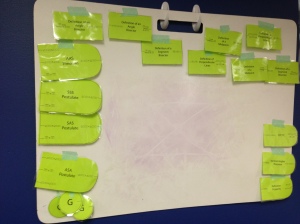
With this in mind, I found out that our community college uses the Accuplacer as a placement test. From there, I looked at the College Boards Accuplacer Program Manual. This document lists math “skills” assessed under 3 categories: Arithmetic, Elementary Algebra & College Level Math. I put all of these skills in a spreadsheet and grouped them by content:

Based on this analysis, I should spend the semester focusing on the following topics:

1. Proportional reasoning
2. solving & graphing equations
3. Systems of equations and inequalities
4. Functions (Linear, Quadratic, Exponential)
5. Solving, graphing, factoring Quadratic Equations

I am confident that the first 3 are skills my students need and I can support their understanding with great modeling tasks and real world applications (and mullets!).

I am struggling with #4 and #5 on the list above for students who are not planning on attending a four year college. This would mean spending weeks on quadratics just to prepare students for a test. I know I can find some good modeling problems, but in all honesty, in my 5 years working a professional civil engineer I did not need to factor any quadratic equations.

My gut instincts and knowledge of these students say to focus on application of exponential functions (credit, interest, population growth, etc) . I also believe that probability and statistics is missing from this list. I want to make curricular decisions based on research, data and experience, not just my “gut instincts”. I also don’t want the class to be a haphazard collection of unrelated tasks.

What would you do for the last six weeks of this class?

Update:

Based on Sandy’s comment below, I looked up our community college score requirements. Here is what I found. Why hadn’t I thought of doing this?!

They are only looking at the Elementary Algebra portion of the Accuplacer! I can filter the spreadsheet above to only show the Elematry Algebra skills and I end up with a much more manageable list. Looking back that the Accuplacer Program Manual (linked above) yeilds this information about the Elementary algebra scores:

Why are system of equations considered to be such an advanced skill?

Whelp, It looks like we will be considering some quadratics. Thank goodness for Algebra Tiles, the Math Assessment Project and Mathalicious!

Painless Proofs!

Last year I didn’t really teach proofs in geometry. It seemed so procedural and I knew it would take time away from more productive problem solving tasks.

I felt guilty for not including proofs in enough detail and I decided that this year I was going to teach it well.

Here is what I did:

1. Our unit began with the Shell Center task: Evaluating Conditions for Congruency, I don’t always follow these lessons exactly as described, but we focused on the Must the Two Triangles be Congruent? part of this lesson. I found that this activity is much smoother and more effective when using AngLegs instead of trying to draw each triangle (described here and here).
2. After this task we formalize our findings in our notebooks documenting which combinations guarantee congruent triangles [SAS, SSS, ASA, AAS] and which do not [SSA, AAA].
3. Next students practice determining if pairs of triangles must be congruent based on the information given. I am careful to include a few with overlapping triangles and triangles who share a side or contain vertical angles to generate observations and class discussions. At this point I address the reflexive property and vertical angles as reasons sides or angles may be congruent. I follow up any student observations of congruency with “How do you know?” or “Explain why you decided these two angles must be the same.”
4. Review definitions of midpoint, bisector, perpendicular, then a few images where I ask if you are given this information, what can you conclude is congruent. See the interactive notes here. This is the day students complete their first few **Really Basic** congruent triangle proofs.
5. Prior to this class I printed Proof Blocks on colored paper and laminated them, then I used masking tape to affix them to  whiteboards. Once class began I randomly assign student pairs and had them work on a wall mounted white board (Vertical non-permanent surface) with a set of Proof Blocks at each work space.

.

Next I project a proof and students copied the image onto the whiteboard, then marked the givens, saw what else they could determine was congruent, and decided if they had enough information to conclude and prove that the triangles must be congruent.

In order to make sure all students got timely feedback and to hold them accountable, I gave each group an index card and when they finished a proof I would check it. Once it was done well, they got a stamp on their card. At the end of class they turned in their index card with their names & stamps on it, similar to the review activity I described earlier.

I returned to this format with the proof blocks and different pairs about once per week after learning and incorporating new skills. The second half of the slides above were from a day after learning and practicing angles formed by parallel lines & a traversal. I intend to create new “blocks” as we advance through the school year. Next week students will prove that all triangles have a sum of 180 degrees and that base angles of isosceles triangles are congruent using the same format.

With students standing at white boards, they can glance around and see their peers work easily, they are more likely to collaborate, and the whiteboard & Proof Blocks make it much easier to adjust their work when there are any corrections required.

I just graded students quizzes over this unit and it is the first time in my 12 year teaching history that they did very well on the first attempt!